Saturation Pressure
For a mixture at given temperature, the pressure at which a new infinitesimal (“incipient”) phase appears upon slight change in pressure. The mixture and its incipient phase are in thermodynamic equilibrium. If the incipient phase is lighter than the mixture phase, the saturation pressure is a bubblepoint and the incipient phase is a bubble appearing from the oil mixture. If the incipient phase is heavier than the mixture phase, the saturation pressure is a dewpoint and the incipient phase is a liquid (“dew”) appearing from the gas mixture. Petroleum mixtures will always exhibit lower dewpoints for the entire range of temperatures exhibiting two phases (i.e. less than the cricondentherm), while upper saturation pressures of both bubblepoint and dewpoint type are usually found in the range of relevant operational temperatures.
1. Generating a Saturation Pressure Calculation
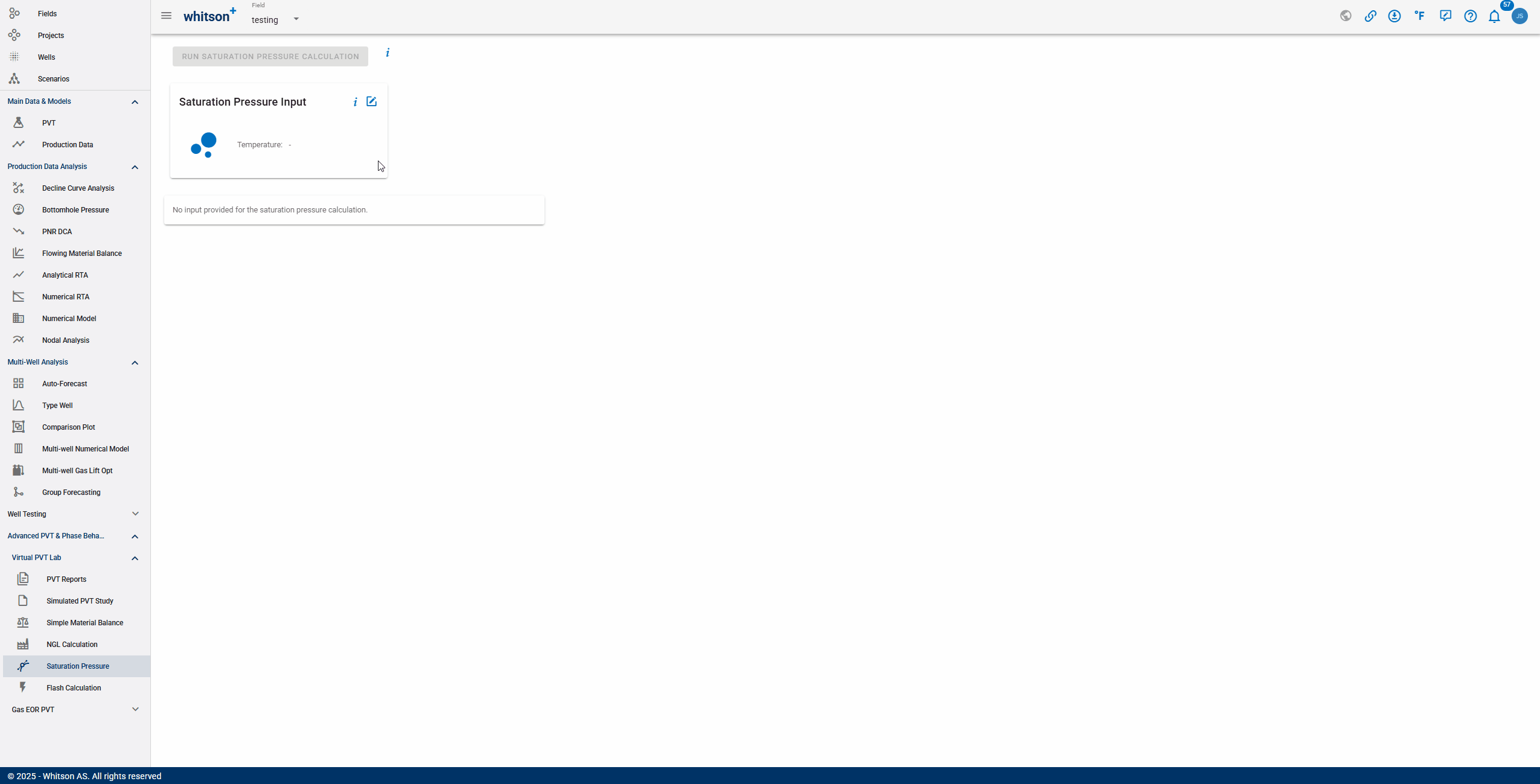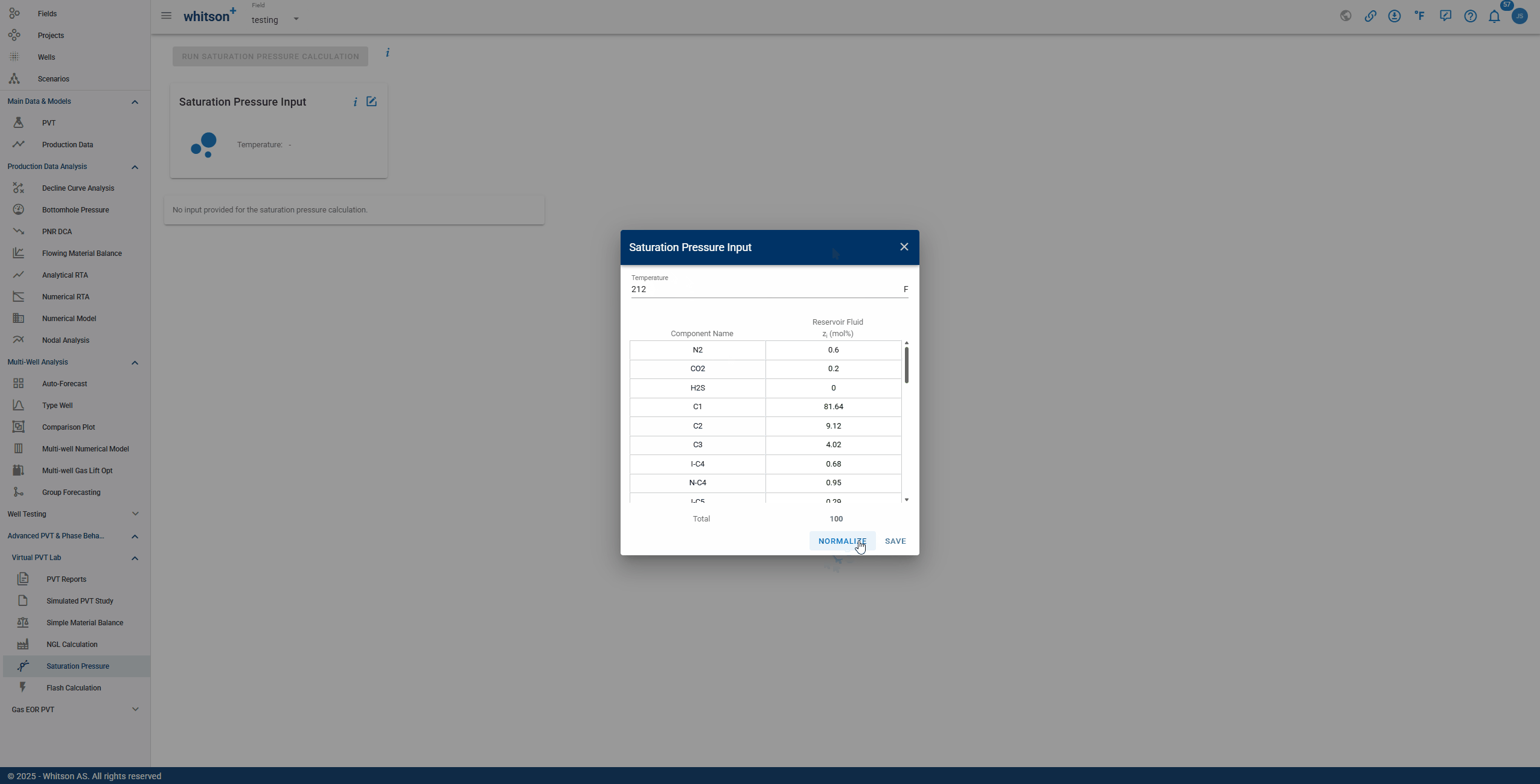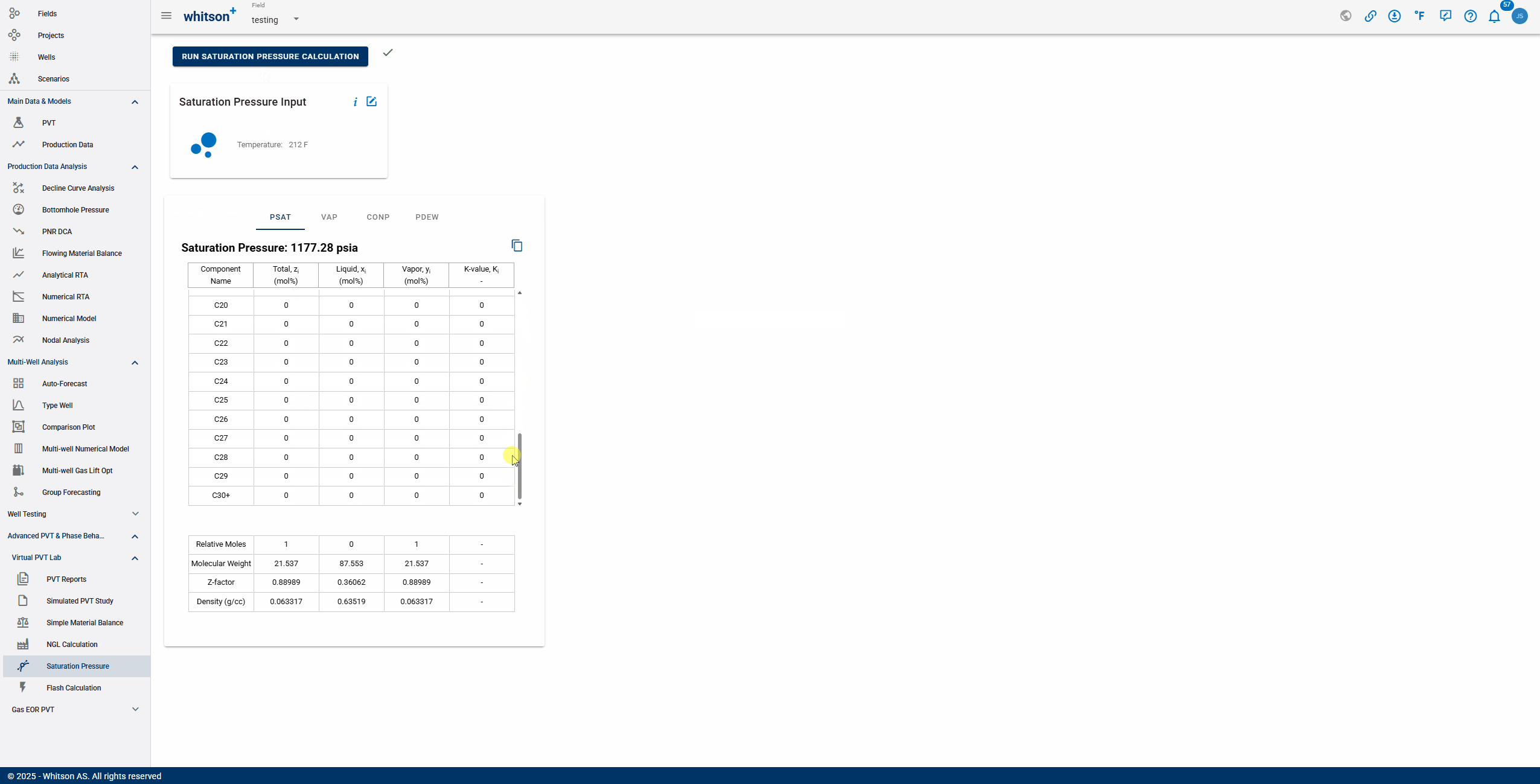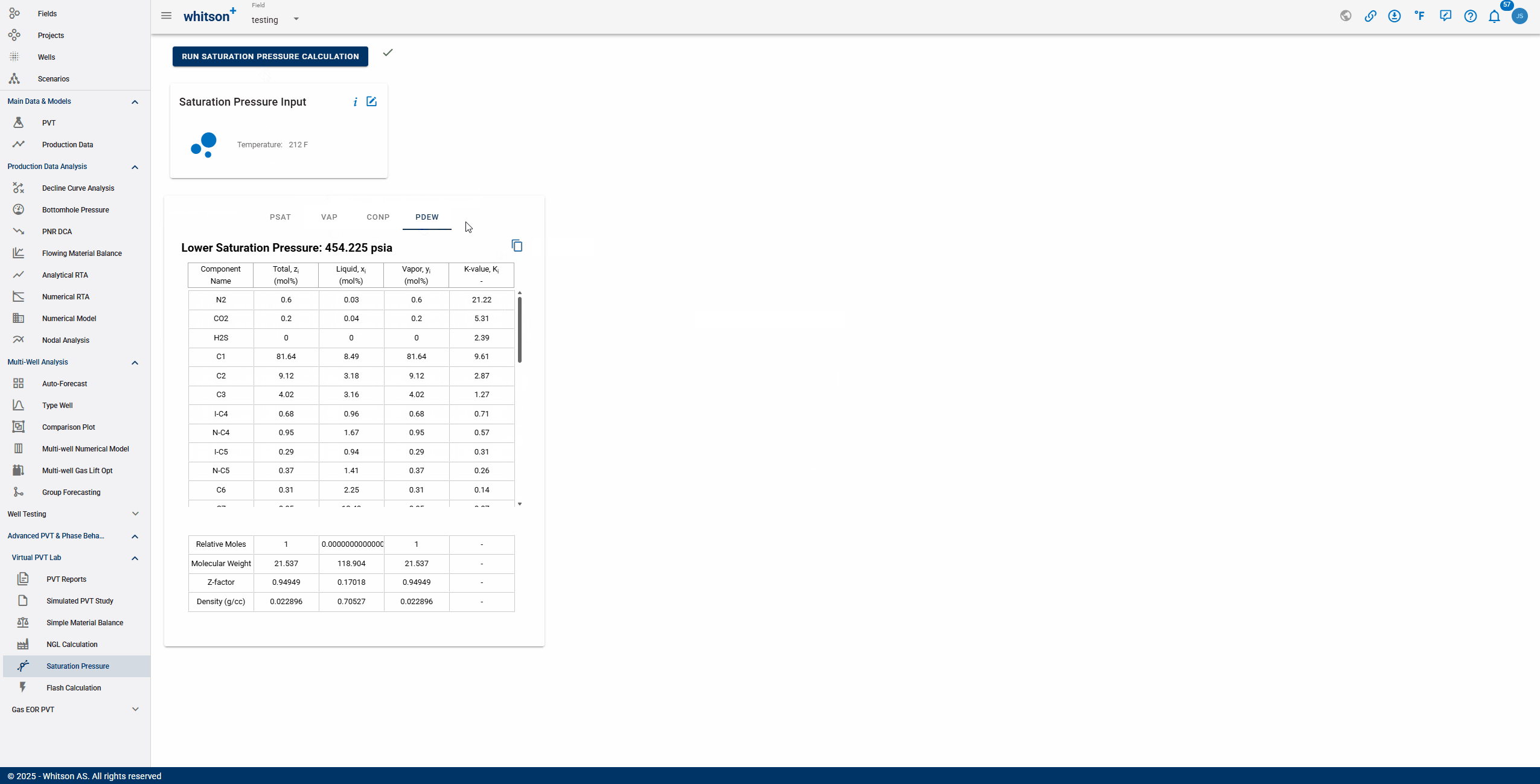
To generate a Saturation Pressure Calculation, enter the temperature at which the components will be tested, along with the composition of each relevant component. The complete process is shown in the GIF below.
Normalization
Ensure the total composition equals 100%. If it does not, click the Normalize button to adjust it automatically. This will allow you to save changes.
2. Terminology
2.1. Bubble-Point
The state of a fluid mixture characterized by the co-existence of a liquid phase saturated with an infinitesimal quantity of equilibrium gas phase.
2.2. Dew-Point
The state of a fluid mixture characterized by the co-existence of a vapor phase saturated with an infinitesimal quantity of equilibrium liquid (condensate) phase.
2.3. Critical-Point
The state of a fluid mixture at which all properties of all coexisting vapor and liquid phases become identical (densities, viscosities, etc.), and the equilibrium ratios \(K_i=1\) for all components. The mixture is called a saturated critical fluid at its critical state (not a saturated bubblepoint oil or a saturated dewpoint gas).
2.4. Vapor Pressure
For a compound at a temperature below the critical temperature (\(T_c\)), down to the triple point \(T_t\), and further down to 0 degrees absolute (\(T_0\)), the vapor pressure defines where the compound exists in a multi-phase thermodynamic equilibrium with (a) saturated vapor and saturated liquid (\(T_t<T<T_c\)), (b) saturated vapor and saturated solid (\(0<T<T_t\)), or (c) saturated vapor, saturated liquid, and saturated solid (\(T=T_t\)). The collection of vapor pressures is called the vapor pressure curve. As temperature increases from the triple point to the critical point, the difference in equilibrium phase properties will decrease monotonically until the phase properties show no difference, and the two phases become identical at the critical point. The Gibbs chemical energy (or fugacities) of saturated phases at the vapor pressure for a given temperature will always be equal, independent of the amount of each equilibrium phase. The system volume will determine how much of each equilibrium phase exists. For a temperature on the saturated vapor-liquid curve (\(T_t<T<T_c\))), the volume changes from its minimum value with 100% saturated liquid to a maximum value with 100% saturated vapor. The pressure will remain completely constant, equal to the vapor pressure, as volume changes from 100% saturated liquid to 100% saturated vapor. A plot of volume versus pressure will, therefore, lead to a horizontal shock line connecting the minimum and maximum saturated volumes. Interestingly, no equation of state functional form reproduces this fundamental (horizontal shock) pressure-volume behavior for any point on the vapor pressure curve (except at the critical point).
2.5. Convergence Pressure
The pressure of a fluid mixture at a given temperature where the K-values of all components appear to converge to unity when the isothermal (\(\log (K_i) - \log (p)\)) curves are extrapolated to pressures above the upper saturation pressure (i.e., into the undersaturated pressure region). The convergence pressure of a mixture can be calculated by an EOS using the negative flash, where the computed equilibrium compositions \(y_i\) and \(x_i\) are identical, fall on a tie line with the original mixture composition, and represent a composition \(z_{ci}=y_i=x_i\) with critical pressure equal to the convergence pressure of \(z_i\) at system temperature.